This is related to the average question.If taking the average is good enough, what about STANDARD DEVIATION?The concept of standard Deviation is more difficult or complicated than other types of concepts.*FROM ALL THE CONCEPTS I KNOW* At this age, we would never use the words, standard deviation but that doesn't mean that we do not try to understand the concept behind it.
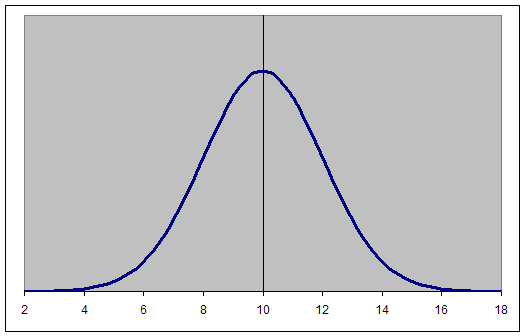
In order to understand this concept,we would first learn about what scientist call normal distribution of data.
It means that most examples in a data are close to average while some examples tends to be extreme.
Example: you are writing a report on nutrition and you need to look at people's daily calorie consumption. Like most data, the numbers for people's typical consumption will turn out to be normally distributed-close to mean while fewer people would tend to overeat or eat less than the mean.
Why is this useful? Here's an example: If you are comparing test scores for different schools, the standard deviation will tell you how diverse the test scores are for each school.
Let's say Springfield Elementary has a higher mean test score than Shelbyville Elementary. Your first reaction might be to say that the kids at Springfield are smarter.
But a bigger standard deviation for one school tells you that there are relatively more kids at that school scoring toward one extreme or the other. By asking a few follow-up questions you might find that, say, Springfield's mean was skewed up because the school district sends all of the gifted education kids to Springfield. Or that Shelbyville's scores were dragged down because students who recently have been "mainstreamed" from special education classes have all been sent to Shelbyville.
